Trading in financial markets is often perceived as a battlefield where traders engage in a constant struggle to outwit each other and emerge victorious. Amidst this competitive landscape, the concept of trading with an “edge” stands out as a crucial determinant of success.
But what exactly does trading with edge mean? In this article, we delve deep into what it is, and how to come up with a trading strategy with an edge.
Understanding “Trading with Edge”
At its core, trading with edge refers to gaining a consistent advantage over the market that leads to profitable outcomes over the long term.
This advantage can stem from various sources, including superior analysis, advanced technology, psychological discipline, or a combination thereof.
Unlike gambling, where outcomes are based on chance, trading with edge involves strategic decision-making grounded in analysis, research, and risk management.
However, there’s a statistical idea we use to figure out if our strategy has an advantage or not, and it’s called expectancy.
What is Expectancy?
Expectancy refers to a statistical concept used to assess the potential profitability or expected return of an investment or trading strategy.
It is a measure that takes into account the probability of different outcomes and their corresponding gains or losses.
The expectancy of an investment or trading strategy is typically calculated by multiplying the probability of each possible outcome by its associated payoff or return, and then summing up these values.
Expectancy = (Probability of Winning * Average Gain) – (Probability of Losing * Average Loss)
Probability of Winning:
This is the likelihood or probability that an investment or trading strategy will result in a profitable outcome. It is often based on historical data, market analysis, or other quantitative or qualitative factors.
Average Gain:
This represents the average amount of profit or gain realized from winning trades or investments. It is calculated by summing up the gains from all winning trades and dividing it by the total number of winning trades.
Probability of Losing:
This is the probability that an investment or trading strategy will result in a loss. It complements the probability of winning, meaning that the sum of the probabilities of winning and losing should equal 1.
Average Loss:
This refers to the average amount of loss incurred from losing trades or investments. It is calculated by summing up the losses from all losing trades and dividing it by the total number of losing trades.
A positive expectancy indicates that, on average, the strategy is expected to generate profits over time, while a negative expectancy suggests the strategy is likely to result in losses.
Therefore, expectancy serves as a useful tool in assessing the effectiveness and risk-reward profile of various investment or trading approaches.
Example:
Let’s simplify things a bit.
Imagine I offered you a game. In this game, I give you two boxes. One box (BOX A) always contains $1, while the other box (BOX B) has a 10% chance of containing $11. and we are going to play this many times.
Throughout the entire game consisting of 10 rounds, you are allowed to select only one box. Once you make your initial choice between BOX A and BOX B, you must stick with that selection for all 10 rounds. In other words, if you choose BOX A initially, you will receive BOX A for all 10 rounds, and if you choose BOX B initially, you will receive BOX B for all 10 rounds.
Therefore, in order to select the box, you cannot simply make a random choice. It is necessary to employ a function or method to calculate and determine the optimal box for your selection. right?
This is where we use expectancy
if you choose BOX A, at the end of the game you may end up with $10, but if you choose BOX B, you can end with $0 or $11
In this case, actually choosing BOX B is better.
So, in every situation, you need to do these calculations when offered different probability games. Let’s calculate them for our example.
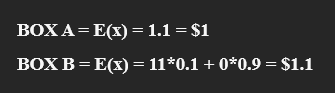
So your expected gain every time you play BOX B is to make $1.1, and for BOX A it’s $1, that’s why if you play this 10 times you end up making $11 with BOX B and $10 with BOX A.
Obviously, if you have a choice, you want to choose the game with a higher expectancy value.
Given that you will be playing this game repeatedly, it is important to note that the probabilities will eventually manifest over time.
Yes, you can choose BOX B and lose all the 10 times and make $0 in the first game. but in the next 10 times, you may win twice or more, so it’s going to average out to the actual probabilities.
Now I have changed the game.
Instead of BOX B having $11, it now has $9, so which one are you going to choose? again to the expectancy.
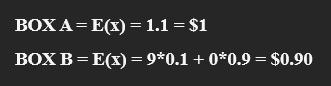
In this case, choosing BOX A is better
What that $0.90 means is that every round you win $0.90 with BOX B and $1 with BOX A so after 10 rounds it’s gonna add up.
In another scenario let’s say BOX B has $10, now which one do you choose?
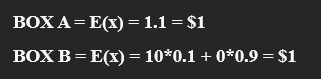
Here both situations give you the same expectancy, but you want to choose BOX A
Because if you have a situation where you can make the same amount of money, you choose the one that is less volatile/less risky.
If you can consistently earn one dollar every time by choosing BOX A, why would you opt for BOX B?, It may provide an average return of one dollar per play but with greater variability in how you earn your money.
Anyways they going to end up converging with each other in the long term.
What does Expectancy have to do with Trading?
Because in any type of game that has to do with probability, it is essential to consider and respect those numbers and never go against the numbers.
Going against the probabilities might result in short-term gains or occasional wins, but in the long run, it is likely to lead to losses.
Example:
Imagine you looking at 2 stocks, STOCK A and STOCK B
STOCK A trading at $20 and it has 60% going up and if it goes up it’s going up to $23, you also think that it has a 40% chance of going down, and if it is going down it’s going all the way to $13.
STOCK B is trading at $10 and it has 40% going up and if it goes up it’s going to $16, on the other hand, it has a 60% chance of going down and it goes down it goes to $8.
Now, which stock will you buy?
Again the expectancy function
In the previous example, I explained it to you with a dollar value, but when we do expectancy for stocks we have to consider the price of stocks, because the price may vary for different stocks so what’s the actual number we want to look is the percentage of gain and lose.
Because if you have a $100 stock that goes from $100 to $105 that’s a 5% return, but if you have a $1 stock that goes up to $1.20 that’s a 20% return.
The first one went up $5 and then 2nd went up 20 cents but a 5% return vs 20% is huge.
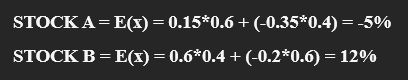
In this situation, you have an expectancy of making 12% if you buy STOCK B and an expectancy of losing -5% if you buy STOCK A.
Even though STOCK B has a 40% going up compared to STOCK A’s 60%, when it goes up you don’t make much money you only make 15%, but when STOCK B goes up you make 60%
And STOCK A when it goes down you lose 35%, but STOCK B when it goes down you lose only 20%. So STOCK B is better.
This is why expectancy is important in trading because we are going to keep repeating, so each time when you play you want to have the highest expectancy number by your side. because it will add up in the long term.
Even if there were no alternative options such as STOCK B available, and you were only presented with the opportunity to buy STOCK A, it would not be advisable to make the purchase. This is because STOCK A has a negative expectancy of -5%, indicating that with each purchase, you would be losing 5% of your investment.
So you wanna have something with positive expectancy and if you have many of them, you choose the one that has the highest.
If you have 2 options with the same expectancy you choose the less volatile one.
To achieve success in trading, it is crucial to adopt a systematic approach. This involves identifying a strategy or method that proves effective and consistently repeating it.
Check out this video for calculating expectancy in real-world trading scenarios
Conclusion
Now that you understand expectancy as the key metric for trading with an edge, how do you ensure you have a strategy with positive expectancy when trading?
Well, in the future, we’ll explore various metrics for trading. There, I’ll explain multiple metrics such as risk-reward ratio, batting average, Sharpe ratio, and more. The only way to have a strategy with positive expectancy, or a way to trade with an edge, is by ensuring all these metrics yield positive results. If they all indicate positive outcomes, then your strategy will indeed have positive expectancy.
